Cut the square with 2 cuts into 4 triangles
Studying geometry in mathematics lessons in grades 5-6
A 1×6 rectangle was cut out of a rectangle of 10×7 cells. as it shown on the picture. Cut the resulting shape into two parts so that they can be folded into a square.
Filled shapes were cut out of a rectangle of 8×9 cells, as shown in the figure. Cut the resulting shape into two equal parts so that they can be folded into a 6×10 rectangle.
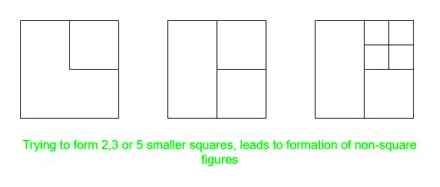
A 5×5 square is drawn on checkered paper. Show how to cut it along the sides of the cells into 7 different rectangles.
Cut a 13×13 square into 5 rectangles along the sides of the cells so that all ten numbers expressing the lengths of the sides of the rectangles are distinct integers.
Divide the shapes shown in the figure into two parts. (You can cut not only along the lines of the cells, but also along their diagonals.)
Cut the shapes shown in the figure into four equal parts.
2.4 Triangle cutting problems
Many scientists have been fond of cutting tasks since ancient times. Solutions to many simple cutting problems were found by the ancient Greeks and Chinese, but the first systematic treatise on this topic belongs to the pen of Abul-Vef, the famous 10th century Persian astronomer who lived in Baghdad. Geometers seriously started solving problems of cutting figures into the smallest number of parts and then making up one or another new figure from them only at the beginning of the twentieth century. One of the founders of this fascinating branch of geometry was the famous puzzle maker Henry E. Dudeny.
Nowadays, puzzle lovers are fond of solving cutting problems primarily because there is no universal method for solving such problems, and everyone who takes on their solution can fully demonstrate their ingenuity, intuition and ability to creative thinking. Since it does not require deep knowledge of geometry, amateurs can sometimes even surpass professional mathematicians.
At the same time, cutting problems are not frivolous or useless, they are not that far from serious mathematical problems.
Cutting tasks help schoolchildren to form geometric representations as early as possible using a variety of materials. When solving such problems, there is a feeling of beauty, law and order in nature.
Is it possible to cut an arbitrary triangle so as to get two triangles?
Marking an angle cut with a speed square
Is it possible to cut a triangle so as to get three triangles?
Is it possible to make two cuts of a triangle to get three triangles?
Is it possible by drawing two cuts of a triangle to get four triangles?
Is it possible to make two cuts of a triangle so as to get five triangles?
How to make two cuts of a triangle to get six triangles?
Is it possible to split a triangle into seven triangles with two cuts?
Is it possible to split a triangle into eight triangles with two cuts?
How many triangles can be obtained when making three cuts of a given triangle?
How many triangles are shown in the picture? Name them.
How many corners do you see in the picture? Name them.
Count how many triangles are shown in the picture?
The chain of tasks is constructed in such a way that when passing to each subsequent figure, the number of required triangles increases (the principle is violated when passing from case “c” to case “r”, but in the case of “r” the “geometric background” becomes more complicated, i.e. such interpenetrating triangles, which consist, for example, of a triangle and a quadrangle, and in the case of “c” all interpenetrating triangles can be considered consisting only of triangles).
1) If the student saw a large triangle consisting of two small ones, i.e. only three triangles, then he gets 1 point.
2) If the student does not see any of the three triangles, then he receives 0 points.
This figure shows a large triangle, consisting of three small, only four triangles. This decision is worth 1 point.
Let’s count all the small triangles, there are only six of them
Let’s count the triangles consisting of two small ones, there are only three of them
Let’s count the triangles, consisting of three small ones, there are only six of them
Triangle made up of six small triangles. 2
1) Students counted (saw) all interpenetrating triangles, they counted using the algorithm. 2 points.
2) The problem was solved without using the algorithm (which triangles the student saw, he counted, but he found more than seven triangles. 1 point).
Framing Square Basics. How to use one
3) The student, when solving, counted less than seven triangles, i.e. did not see interpenetrating triangles. score 0 points.
1) Let’s count the triangles in the “lower” part of the figure, there are only six of them, and they all consist only of triangles.
2) Add the “top” part, we get triangles consisting of triangles and a quadrangle.
It turned out in total: (321) (321) = 12 triangles.
1) The student has counted all triangles using the algorithm (the choice of the algorithm does not matter). score 3 points.
2) The student applied an algorithm for the solution that does not allow to select all the triangles in the figure. score 2 points.
3) Students who do not see interpenetrating triangles receive 1 point.
4) Students who see less than seven triangles in the picture receive 0 points.
Split Square Into Equal Parts || 6th Grade Logical Puzzle || Solution Explained
Count the number of triangles shown in the picture.